Prime Numbers: FAQs & Checker
Prime Number Checker
Core Concepts of Prime Numbers
What is a prime number? (Covers: "what is meant by prime number", "what's a prime number", "what is prime number", "what is a prime number definition", "what is a prime number in math", "what is a prime number maths", "a prime number", "what a prime number", "what is the definition of a prime number", "what makes a number prime")
A prime number is a natural number (a positive whole number: 1, 2, 3, ...) greater than 1 that has exactly two distinct positive divisors: 1 and itself.
Let's break that down:
- Natural number: Prime numbers are positive whole numbers. This excludes negative numbers, fractions, and decimals.
- Greater than 1: The number 1 is not considered a prime number (more on this later).
- Exactly two distinct positive divisors:
- A "divisor" (or factor) of a number is a whole number that divides it exactly, leaving no remainder.
- For a number to be prime, it must be divisible by 1, and it must be divisible by itself.
- Crucially, these two divisors (1 and the number itself) must be the *only* positive divisors. For example, the number 2 has divisors 1 and 2. The number 3 has divisors 1 and 3. The number 5 has divisors 1 and 5.
Numbers that are greater than 1 and are not prime are called composite numbers. A composite number has more than two distinct positive divisors. For example, 4 is composite because its divisors are 1, 2, and 4. The number 6 is composite because its divisors are 1, 2, 3, and 6.
The first few prime numbers are: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, ...
Is 1 a prime number? (Covers: "is one a prime number", "why is 1 not a prime number", "is number 1 a prime number", "why 1 not a prime number", "is 1 a prime number or a composite number", "is 1 a prime or composite number", "is 1 prime number", "why is one not a prime number", "is 1 a composite or prime number")
No, 1 is not a prime number.
By the definition, a prime number must have exactly two distinct positive divisors. The number 1 only has one positive divisor: itself (1). Since it doesn't have two distinct positive divisors, it doesn't fit the definition of a prime number.
Furthermore, 1 is also not a composite number. A composite number must have more than two distinct positive divisors. Since 1 only has one, it's not composite either.
Thus, the number 1 is in a special category of its own – it is called a unit.
Why is this distinction important? One of the main reasons is the Fundamental Theorem of Arithmetic. This theorem states that every integer greater than 1 either is a prime number itself or can be represented as a product of prime numbers, and this factorization is unique (apart from the order of the factors). If 1 were considered prime, this uniqueness would be lost. For example, if 1 were prime, we could write:
- 6 = 2 × 3
- 6 = 1 × 2 × 3
- 6 = 1 × 1 × 2 × 3
...and so on, leading to an infinite number of "prime" factorizations for any number. Excluding 1 from the primes ensures that prime factorization is unique, which is fundamental to many areas of number theory.
Is 0 a prime number? (Covers: "is zero a prime number", "is 0 prime number")
No, 0 is not a prime number.
There are several reasons why:
- Not greater than 1: The definition of a prime number explicitly states it must be a natural number greater than 1. Zero does not meet this criterion.
- Infinite divisors: Zero is divisible by every non-zero integer (e.g., 0 ÷ 5 = 0, 0 ÷ -10 = 0). Prime numbers must have exactly two distinct positive divisors. Zero has an infinite number of divisors, so it doesn't fit this part of the definition either.
Is 2 a prime number? (Covers: "is two a prime number")
Yes, 2 is a prime number.
- It is a natural number greater than 1.
- Its only positive divisors are 1 and 2 (exactly two distinct positive divisors).
The number 2 is special because it is the smallest prime number and the only even prime number. Any other even number greater than 2 is divisible by 2 (in addition to 1 and itself), so it will have at least three divisors and thus be composite (e.g., 4 is divisible by 1, 2, and 4).
Specific Number Checks
Here's a quick rundown for other common number queries. The general method to check is to see if the number has any divisors other than 1 and itself. You only need to check for divisibility by prime numbers up to the square root of the number.
Is 3 a prime number? (Covers: "is three a prime number")
Yes, 3 is a prime number. Its only positive divisors are 1 and 3.
Is 4 a prime number?
No, 4 is not a prime number. It is a composite number. Its positive divisors are 1, 2, and 4.
Is 5 a prime number? (Covers: "is five a prime number")
Yes, 5 is a prime number. Its only positive divisors are 1 and 5.
Is 6 a prime number?
No, 6 is not a prime number. It is a composite number. Its positive divisors are 1, 2, 3, and 6.
Is 7 a prime number?
Yes, 7 is a prime number. Its only positive divisors are 1 and 7.
Is 8 a prime number?
No, 8 is not a prime number. It is a composite number. Its positive divisors are 1, 2, 4, and 8.
Is 9 a prime number? (Covers: "is nine a prime number")
No, 9 is not a prime number. It is a composite number. Its positive divisors are 1, 3, and 9.
Is 10 a prime number?
No, 10 is not a prime number. It is a composite number. Its positive divisors are 1, 2, 5, and 10.
Is 11 a prime number?
Yes, 11 is a prime number. Its only positive divisors are 1 and 11.
Is 12 a prime number?
No, 12 is not a prime number. It is a composite number. Its positive divisors are 1, 2, 3, 4, 6, and 12.
Is 13 a prime number?
Yes, 13 is a prime number. Its only positive divisors are 1 and 13.
Is 15 a prime number?
No, 15 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 5, and 15.
Is 16 a prime number?
No, 16 is not a prime number. It is a composite number. Its positive divisors are 1, 2, 4, 8, and 16.
Is 17 a prime number?
Yes, 17 is a prime number. Its only positive divisors are 1 and 17.
Is 19 a prime number? (Covers: "is 19 prime number")
Yes, 19 is a prime number. Its only positive divisors are 1 and 19.
Is 21 a prime number?
No, 21 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 7, and 21 (since 3 × 7 = 21).
Is 23 a prime number? (Covers: "is 23 prime number")
Yes, 23 is a prime number. Its only positive divisors are 1 and 23.
Is 25 a prime number?
No, 25 is not a prime number. It is a composite number. Its positive divisors are 1, 5, and 25 (since 5 × 5 = 25).
Is 27 a prime number?
No, 27 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 9, and 27 (since 3 × 9 = 27).
Is 29 a prime number?
Yes, 29 is a prime number. Its only positive divisors are 1 and 29.
Is 31 a prime number?
Yes, 31 is a prime number. Its only positive divisors are 1 and 31.
Is 33 a prime number?
No, 33 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 11, and 33 (since 3 × 11 = 33).
Is 35 a prime number?
No, 35 is not a prime number. It is a composite number. Its positive divisors are 1, 5, 7, and 35 (since 5 × 7 = 35).
Is 37 a prime number?
Yes, 37 is a prime number. Its only positive divisors are 1 and 37.
Is 39 a prime number?
No, 39 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 13, and 39 (since 3 × 13 = 39).
Is 41 a prime number? (Covers: "is 41 prime number")
Yes, 41 is a prime number. Its only positive divisors are 1 and 41.
Is 43 a prime number?
Yes, 43 is a prime number. Its only positive divisors are 1 and 43.
Is 47 a prime number?
Yes, 47 is a prime number. Its only positive divisors are 1 and 47.
Is 49 a prime number?
No, 49 is not a prime number. It is a composite number. Its positive divisors are 1, 7, and 49 (since 7 × 7 = 49).
Is 51 a prime number? (Covers: "is 51 prime number")
No, 51 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 17, and 51 (since 3 × 17 = 51).
Is 53 a prime number?
Yes, 53 is a prime number. Its only positive divisors are 1 and 53.
Is 57 a prime number?
No, 57 is not a prime number. It is a composite number. Its positive divisors are 1, 3, 19, and 57 (since 3 × 19 = 57).
Is 59 a prime number?
Yes, 59 is a prime number. Its only positive divisors are 1 and 59.
Is 61 a prime number? (Covers: "is 61 prime number")
Yes, 61 is a prime number. Its only positive divisors are 1 and 61.
Is 63 a prime number?
No, 63 is not a prime number. It is a composite number. For example, 3 × 21 = 63, or 7 × 9 = 63. Its positive divisors are 1, 3, 7, 9, 21, and 63.
Is 67 a prime number?
Yes, 67 is a prime number. Its only positive divisors are 1 and 67.
Is 69 a prime number?
No, 69 is not a prime number. It is a composite number. For example, 3 × 23 = 69. Its positive divisors are 1, 3, 23, and 69.
Is 71 a prime number?
Yes, 71 is a prime number. Its only positive divisors are 1 and 71.
Is 73 a prime number?
Yes, 73 is a prime number. Its only positive divisors are 1 and 73.
Is 79 a prime number?
Yes, 79 is a prime number. Its only positive divisors are 1 and 79.
Is 81 a prime number?
No, 81 is not a prime number. It is a composite number. For example, 9 × 9 = 81. Its positive divisors are 1, 3, 9, 27, and 81.
Is 83 a prime number?
Yes, 83 is a prime number. Its only positive divisors are 1 and 83.
Is 87 a prime number?
No, 87 is not a prime number. It is a composite number. For example, 3 × 29 = 87. Its positive divisors are 1, 3, 29, and 87.
Is 89 a prime number?
Yes, 89 is a prime number. Its only positive divisors are 1 and 89.
Is 91 a prime number?
No, 91 is not a prime number. It is a composite number. It's often mistaken for a prime, but 7 × 13 = 91. Its positive divisors are 1, 7, 13, and 91.
Is 93 a prime number?
No, 93 is not a prime number. It is a composite number. For example, 3 × 31 = 93. Its positive divisors are 1, 3, 31, and 93.
Is 97 a prime number?
Yes, 97 is a prime number. Its only positive divisors are 1 and 97. It is the largest prime number less than 100.
Is 101 a prime number?
Yes, 101 is a prime number. Its only positive divisors are 1 and 101.
Is 111 a prime number?
No, 111 is not a prime number. It is a composite number. The sum of its digits (1+1+1=3) is divisible by 3, so 111 is divisible by 3 (111 = 3 × 37). Its positive divisors are 1, 3, 37, and 111.
Is 113 a prime number?
Yes, 113 is a prime number. Its only positive divisors are 1 and 113.
Is 117 a prime number?
No, 117 is not a prime number. It is a composite number. The sum of its digits (1+1+7=9) is divisible by 9 (and 3), so 117 is divisible by 9 (117 = 9 × 13 or 3 x 39). Its positive divisors include 1, 3, 9, 13, 39, and 117.
Is 119 a prime number?
No, 119 is not a prime number. It is a composite number. It can be factored as 7 × 17 = 119. Its positive divisors are 1, 7, 17, and 119.
Is 121 a prime number?
No, 121 is not a prime number. It is a composite number. It is a perfect square: 11 × 11 = 121. Its positive divisors are 1, 11, and 121.
Is 127 a prime number?
Yes, 127 is a prime number. Its only positive divisors are 1 and 127. (It's a Mersenne prime, 27-1).
Advanced Topics
How to check if a number is prime? (Covers: "how to find if a number is prime", "how to know if a number is prime", "how to tell if a number is prime", "how do you find the prime number", "how to check if number is prime", "how to check whether a number is prime")
To determine if a number n is prime, you can use the trial division method:
- Handle base cases:
- If
n ≤ 1, it is not prime. - If
n = 2, it is prime (the only even prime). - If
n > 2andnis even (i.e.,n % 2 == 0), it is not prime.
- If
- Iterate through odd divisors:
If
nis odd and greater than 2, you only need to check for divisibility by odd numbers from 3 up to the square root ofn(√n).Why
√n? Ifnhas a divisor larger than√n, sayd1, thenn = d1 × d2. Ifd1 > √n, thend2must be less than√n(otherwised1 × d2 > n). So, if a divisor exists, one of them must be less than or equal to√n. If we don't find any divisors up to√n, there won't be any larger ones either (exceptnitself). - Check for divisibility:
In each step of the iteration (for an odd number
ifrom 3 up to√n), check ifn % i == 0(i.e., ifnis divisible byiwith no remainder).- If you find such a divisor
i, thennis not prime, and you can stop.
- If you find such a divisor
- Conclusion:
If you go through all the checks up to
√nand find no divisors, thennis prime.
The calculator provided on this page uses this logic.
What is the smallest prime number?
The smallest prime number is 2.
As discussed earlier, 1 is not prime. 2 fits the definition: it's greater than 1 and its only positive divisors are 1 and 2.
What is the largest prime number? (Covers: "what is the biggest prime number", "what is the last prime number", "what is the highest prime number")
There is no largest prime number.
This was proven by the ancient Greek mathematician Euclid around 300 BC. His proof (Euclid's Theorem) essentially shows that if you assume there is a largest prime number, you can always construct another prime number larger than it, which leads to a contradiction. Therefore, the set of prime numbers is infinite.
However, people often talk about the "largest known prime number." These are usually Mersenne primes (primes of the form 2p - 1, where p is also prime). As of my last update, the largest known prime number is 282,589,933 − 1, a number with 24,862,048 digits, discovered in December 2018 by the Great Internet Mersenne Prime Search (GIMPS). New, larger primes are occasionally found as computational power increases.
On a scale of 7, 13 or 31 how much do you love prime numbers? Why is one not a prime number? What makes two the only even prime number? In this article we discuss the answers to these questions and learn about the fascinating properties of prime numbers.
Key takeaways
- A prime number is a whole number with exactly two factors: one and itself.
- The number one has only one factor and a prime number must have precisely two factors. Only having one factor is why one is not a prime number.
- Arrays can be used to illustrate the factors of a number.
What are prime numbers in maths?
Prime numbers are positive integers with exactly two distinct positive factors. The two factors of a prime are always one and the number itself. For example, seven is a prime number because the only factors are one and seven.
If a number has more than two factors, it is a composite number. For example, eight is a composite number because the factors are one, two, four and eight. The first, and smallest, prime number is two.
Prime numbers one to 100
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97
Even prime numbers
As we mentioned, two is a prime number because it has exactly two factors, one and two. Besides being the first prime, two is the only even prime number. All other even numbers have two as a factor, making them composite numbers.
How do you figure out if a number is prime?
To determine if a number is prime, list all its factors. If the number has exactly two factors, then it is prime. If a number has more than two factors it is a composite number.
Representing a prime number
Concrete, pictorial and abstract representations are an important part of developing a conceptual understanding of prime numbers. Arrays are a useful representation to illustrate the factors of a number.
Prime numbers only have one array representation – this is a single line of counters. The array can be horizontal, vertical or any orientation. It is the dimensions of the array that illustrate the factors of a number. A single line of counters represent one as a factor and the number itself.
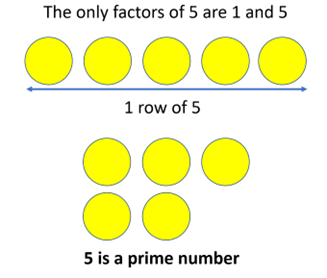
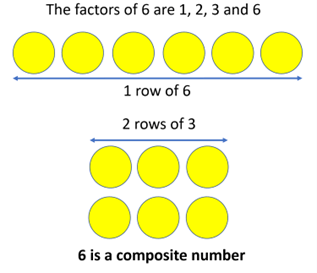
Five is prime as it only has one array, whereas six is a composite number since it has two or more arrays.
In Year 7, we explore arrays of numbers using counters in the Prime numbers & proof block.
What are the rules for prime numbers?
- Prime numbers are integers greater than one with exactly two distinct positive factors.
- The only even prime is two; all other even numbers are composite.
- Prime numbers greater than two are odd.
What is the fastest way to find prime numbers?
To find prime numbers, identify all the factors of a number. An efficient way is using factor pairs. Here are the first 10 positive integers with their factor pairs:
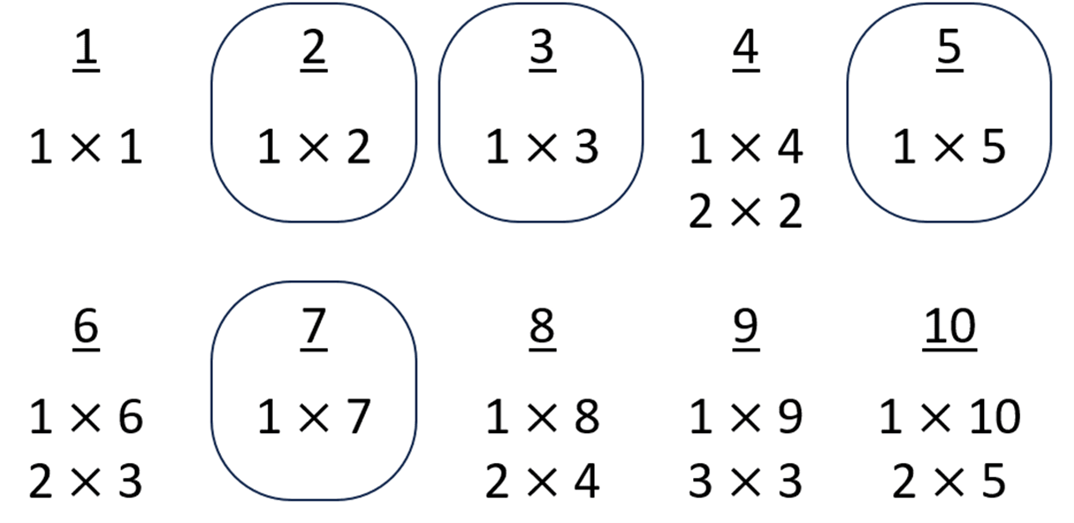
Be aware, factor pairs can cause misconceptions to arise. Here, one can be mistaken for having exactly two factors. However, notice that one is being multiplied by itself – making it the only factor. Since it has only one factor, one is not prime.
Notice also, four and nine only have three factors. Representing four as two rows of two counters illustrates that four is a square number, just like nine.
Are prime numbers hard to find?
As numbers increase, there are fewer prime numbers, this makes them harder to find. Rules of divisibility can be employed to identify primes more efficiently. Here are some examples:
7,314: Ends in a four so is an even number, meaning two is a factor. Therefore, 7,314 is not prime.
8,455: Ends in a five so is a multiple of five, meaning five is a factor. Therefore, 8,455 is not prime.
1,263: 1 + 2 + 6 + 3 = 12, 12 has three as a factor meaning 1,263 also has three as a factor. Therefore, 1,263 is not prime.
This reductive reasoning can make it easier to find prime numbers.
What is the best way to find a prime number?
One of the oldest and, to this day, most efficient methods for finding prime numbers up to a certain number, n, is to iteratively list all the multiples of each prime number. Then the unmarked values are primes.
We know this doesn’t feel fast, but it’s the best way to look for primes!
Some primes have certain patterns or special properties that can help identify them efficiently:
While there are many methods to find or identify prime numbers, for extremely large numbers it can be difficult for anyone to determine with absolute certainty.
Why one is not a prime number
One is not a prime number because of the role prime numbers play in the fundamental structure of mathematics. Primes are the building blocks of natural numbers; every positive integer can be uniquely expressed as a product of primes – this is known as prime factorization.
For example:
How do you prove one is not prime?
The proof that one is not prime lies in their definition. By definition, prime numbers are positive integers with exactly two factors: one and the number itself. Since one has only one factor – itself – it fails to meet the fundamental criteria.
Another perspective on this is that prime numbers are those that are divisible only by one and themselves.
As one is the exception in having only one factor, it is excluded from the category of prime numbers. This proof aligns with the conventional definition and properties of prime numbers in mathematics.
Example prime number questions
- Which number is the only even prime number?
- When you add together two prime numbers, do they always give an even number? Explain your answer.
- Which large numbers can you tell are not prime just by looking at their digits?
- You’re playing a raffle game, with tickets numbered 1 to 100. You can win a prize if you pick a ticket that meets one of these two criteria:
- Win a prize for a multiple of eight.
- Win a prize for a prime number.
Which criteria would you choose to have a better chance of winning? Explain why
In summary
Understanding the significance of prime numbers illuminates fundamental principles in mathematics. Through their definition as integers with precisely two factors, primes serve as the building blocks of natural numbers, essential for prime factorization. This underlines why one, with its sole factor, does not qualify as prime.
Meanwhile, exploring the properties and patterns of prime numbers enriches mathematical insight, from discerning Mersenne primes to the hunt for twin primes. While strategies exist for identifying primes, the inherent complexity of larger numbers stresses their challenging nature. Thus, exploring prime numbers unveils both the elegance and complex nature inherent in mathematics.