Relative Frequency Calculator
How to Calculate Relative Frequency
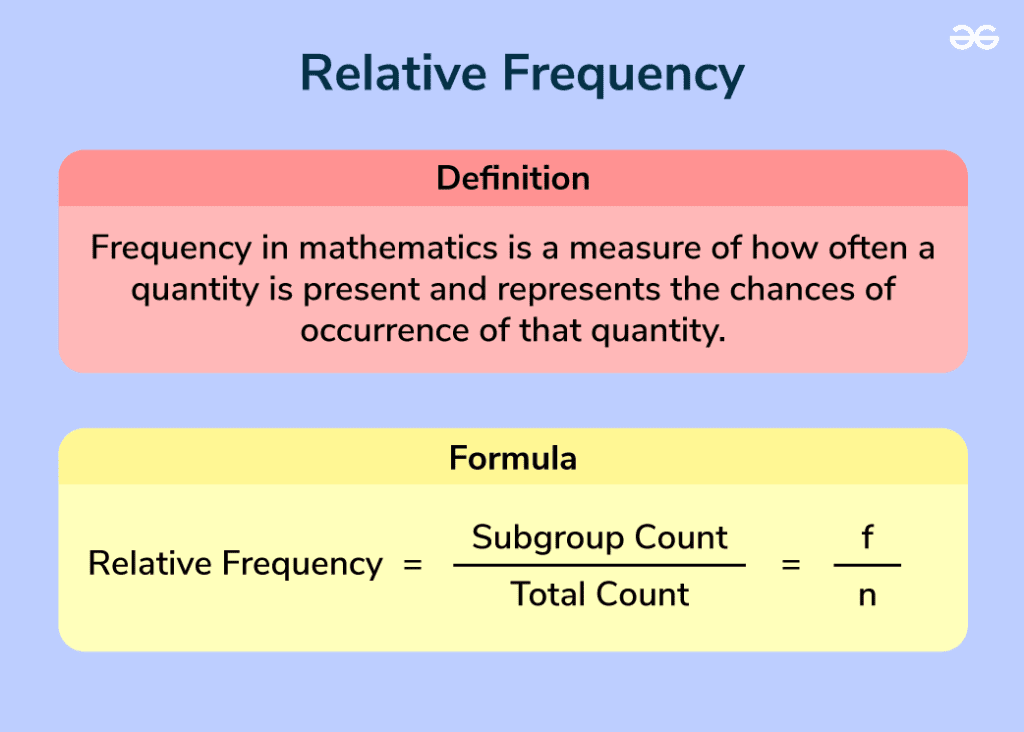
Relative frequency is a measure of how often a particular event occurs in relation to the total number of events or observations. It is a simple way to understand the likelihood or proportion of a specific event occurring within a dataset.
Formula for Relative Frequency:
Relative Frequency = (Frequency of the Event / Total Number of Observations)
Step-by-Step Guide to Calculate Relative Frequency
Here’s a step-by-step guide to calculate relative frequency:
Step 1: Determine the Frequency of the Event
The first step is to determine how often the event occurred. For example, if a specific outcome appeared 15 times out of 100 total observations, the frequency is 15.
Step 2: Determine the Total Number of Observations
The next step is to calculate the total number of observations. This could be the total number of events, experiments, or instances recorded.
Step 3: Divide the Frequency by the Total Number of Observations
To find the relative frequency, divide the frequency of the event by the total number of observations.
Step 4: Interpret the Result
The resulting number is the relative frequency. It will typically be a decimal between 0 and 1, which can also be expressed as a percentage. For example, if the relative frequency is 0.15, you can say that the event occurred 15% of the time in the dataset.
Why is Relative Frequency Important?
Relative frequency provides an easy way to visualize the likelihood of an event and compare it with other outcomes. It is often used in statistics to summarize categorical data and is especially useful when dealing with large datasets.
Relative frequency is a key concept in statistics that helps in understanding how often a particular value occurs in comparison to the total number of observations. Whether you are studying statistics or simply analyzing data for a project, learning how to calculate relative frequency can provide valuable insights. This blog post will guide you through the steps to calculate relative frequency, explain its significance, and answer frequently asked questions to help you grasp this concept thoroughly.
What is Relative Frequency?
Relative frequency is the ratio of the number of times a specific event occurs to the total number of observations in a data set. It provides a way to express how often an event happens relative to the total occurrences, usually represented as a percentage or a fraction.
The formula for relative frequency is:
Step-by-Step Guide to Calculate Relative Frequency
Step 1: Determine the Frequency of the Event
The first step in calculating relative frequency is to determine how often a specific event occurs in the data set. This is known as the frequency of the event.
Example: Suppose you have a data set of students’ favorite fruits, and you want to calculate the relative frequency of students who prefer apples.
Data Set: [Apple, Banana, Apple, Orange, Apple, Banana]
Frequency of Apple = 3
Step 2: Calculate the Total Number of Observations
Next, determine the total number of observations in the data set.
Example:
Total Number of Observations = 6 (since there are 6 students in the data set)
Step 3: Calculate Relative Frequency
To calculate relative frequency, divide the frequency of the event by the total number of observations.
Example:
Relative Frequency of Apple = or 50%
Final Answer: The relative frequency of students who prefer apples is 0.5 or 50%.
Importance of Relative Frequency
Understand Data Distribution: Relative frequency helps in understanding how often certain values occur within a data set, which is useful for identifying trends and patterns.
Comparison: It allows for easy comparison between different categories, as it normalizes the data, making it easier to interpret.
Probability Estimation: In statistics, relative frequency is often used as an estimate of the probability of an event occurring.
Relative Frequency Table
A relative frequency table is a useful way to organize data to show the relative frequency of each value or category. It is particularly helpful when working with large data sets.
Example: Consider a survey that records the favorite color of 10 people:
Red: 3
Blue: 4
Green: 3
| Color | Frequency | Relative Frequency |
|---|---|---|
| Red | 3 | or 30% |
| Blue | 4 | or 40% |
| Green | 3 | or 30% |
Examples of Calculating Relative Frequency
Example 1: In a dice-rolling experiment, you roll a die 20 times and get the number 4 five times.
Frequency of getting 4 = 5
Total Number of Rolls = 20
Relative Frequency = or 25%
Example 2: You conduct a survey of 50 people to find out their preferred mode of transportation. 20 people say they prefer cars.
Frequency of Cars = 20
Total Number of Respondents = 50
Relative Frequency = or 40%
Frequently Asked Questions about Relative Frequency
Q1: What is the formula for calculating relative frequency?
The formula for relative frequency is:
Q2: How is relative frequency different from frequency?
Frequency refers to the number of times an event occurs, while relative frequency is the ratio of the frequency to the total number of observations, often expressed as a percentage or fraction.
Q3: What is a relative frequency table?
A relative frequency table is a table that shows the frequency of each category along with its relative frequency, helping to visualize the distribution of data.
Q4: Why is relative frequency important?
Relative frequency is important because it allows you to understand how often an event occurs in relation to the entire data set, making it easier to compare different events or categories.
Q5: Can relative frequency be greater than 1?
No, relative frequency cannot be greater than 1. It is a ratio that represents a part of the whole, so it ranges from 0 to 1, or 0% to 100%.
Q6: How do you interpret relative frequency?
Relative frequency is interpreted as the proportion of times an event occurs compared to the total number of observations. For example, a relative frequency of 0.25 means the event occurs 25% of the time.
Q7: How is relative frequency used to estimate probability?
Relative frequency can be used as an estimate of the probability of an event occurring, especially when dealing with empirical data. For example, if an event occurs 30 times out of 100 trials, the estimated probability is 0.3 or 30%.
Q8: What is cumulative relative frequency?
Cumulative relative frequency is the sum of the relative frequencies for all values less than or equal to a given value. It helps in understanding the cumulative distribution of data.
Q9: How do you create a relative frequency histogram?
A relative frequency histogram is created by plotting the relative frequencies of different categories or intervals on the y-axis, with the categories or intervals on the x-axis. It is useful for visualizing data distribution.
Q10: Can relative frequency be negative?
No, relative frequency cannot be negative. It represents a proportion of occurrences, so it ranges from 0 to 1.
Conclusion
Calculating relative frequency is a fundamental skill in statistics that helps in understanding how often a particular event occurs in comparison to the total data set. By using the simple formula of dividing the event frequency by the total number of observations, you can easily determine the relative frequency and gain valuable insights into your data. Whether you’re analyzing survey results, conducting experiments, or studying data trends, understanding relative frequency will help you interpret and present your data effectively.
Now that you know how to calculate relative frequency, try using it in your own data analysis projects to better understand patterns and trends.
