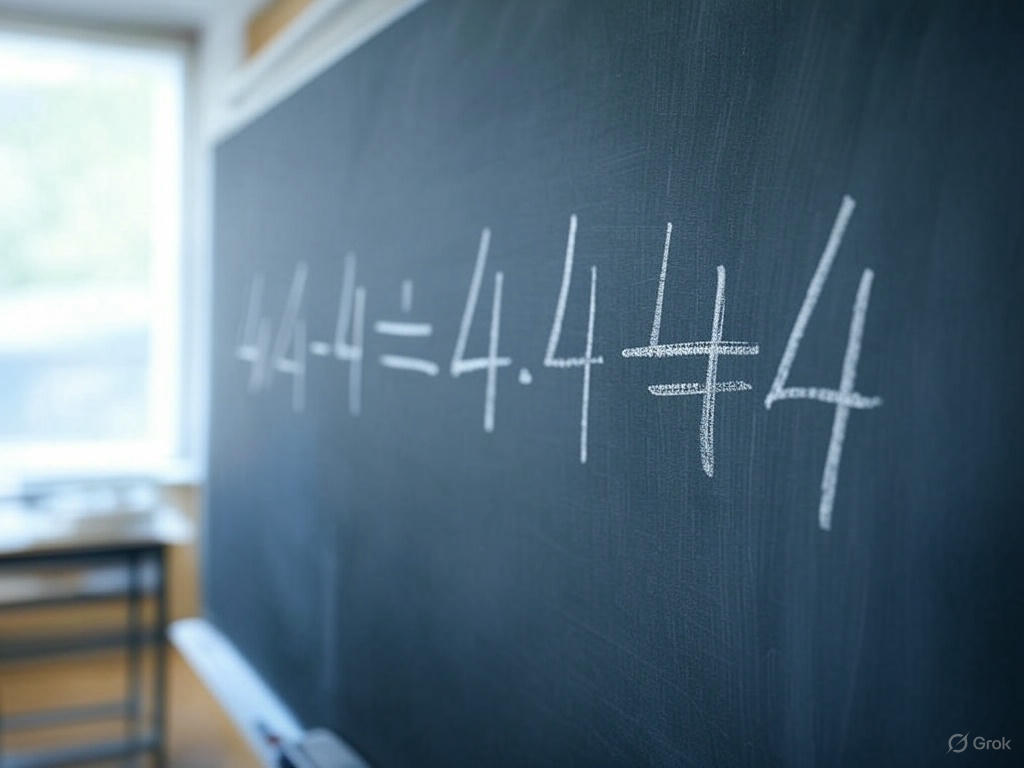The Hardest Easy Math Problem: The 4×4-4÷4+4 Challenge
Introduction: When Simple Math Becomes Controversial
Math is often seen as the epitome of certainty—a realm where questions have definitive answers. Yet, occasionally, seemingly elementary problems spark heated debates across the internet, dividing friends, families, and even mathematicians. The 4×4-4÷4+4 challenge is one such mathematical puzzle that, despite its innocent appearance, has caused tremendous controversy online.
What makes this problem the “hardest easy” math challenge? It’s deceptively simple: just four 4s connected by basic arithmetic operations. No advanced calculus, no complex algebraic equations—just multiplication, division, subtraction, and addition with a single-digit number. Yet this humble expression has led millions of people to different answers, igniting passionate arguments about who’s right and who’s wrong.
In this comprehensive exploration, we’ll dive deep into the 4×4-4÷4+4 challenge, unraveling why it confuses so many people, exploring the critical role of order of operations, and examining why such elementary math problems frequently go viral. Whether you’re a math enthusiast, a confused participant in an online debate, or simply curious about why people fight over arithmetic, this guide will provide clarity and insight into one of the internet’s most divisive math problems.
Understanding the 4×4-4÷4+4 Challenge
What Exactly Is the Problem?
The challenge presents itself innocuously: calculate the value of 4×4-4÷4+4.
At first glance, this looks straightforward. We have four instances of the number 4, connected by three basic operations: multiplication (×), division (÷), subtraction (-), and addition (+). Anyone with elementary school math education should be able to solve this, right?
Yet when this problem circulates on social media platforms like Facebook, Twitter, or Instagram, the comment sections invariably explode with conflicting answers. Some confidently declare the answer is 17, others insist it’s 16, while still others argue for 15 or even 1.
The Problem Goes Viral
The 4×4-4÷4+4 challenge gained significant traction around 2019, joining the ranks of other viral math problems like “8÷2(2+2)” that divide internet users into passionate camps. These problems spread rapidly because they hit a perfect sweet spot: they appear simple enough that everyone feels qualified to answer, yet they contain enough complexity to generate legitimate confusion.
When celebrities, influencers, and media outlets share these problems, they often receive millions of interactions. A single post posing the 4×4-4÷4+4 challenge might generate thousands of comments from people absolutely certain of their contradictory answers, each wondering how others could be so “obviously wrong.”
Why Such Confusion?
The confusion stems from several factors:
- Ambiguous notation: The expression 4×4-4÷4+4 uses a mix of operation symbols that can be interpreted differently depending on how you read them
- Order of operations uncertainty: Many people learned different acronyms (PEMDAS, BODMAS, etc.) or have forgotten the precise rules
- Calculator discrepancies: Different calculators might give different answers depending on their programming
- Educational variations: The emphasis placed on order of operations varies in different educational systems worldwide
This perfect storm of ambiguity creates an environment where intelligent people can arrive at different answers while following what they believe are correct mathematical principles.
The Critical Importance of Order of Operations
What Is the Order of Operations?
The order of operations is a convention in mathematics that establishes the sequence in which operations should be performed in an expression with multiple operations. Without such a convention, expressions like 4×4-4÷4+4 would be ambiguous, with multiple possible interpretations.
The standard order of operations is commonly taught using acronyms that help students remember the sequence:
- PEMDAS: Parentheses, Exponents, Multiplication/Division (from left to right), Addition/Subtraction (from left to right)
- BODMAS: Brackets, Orders (powers/roots), Division/Multiplication (from left to right), Addition/Subtraction (from left to right)
- BEDMAS: Brackets, Exponents, Division/Multiplication (from left to right), Addition/Subtraction (from left to right)
Despite the differences in these acronyms, they all describe the same conventional order. The key point many people miss is that multiplication and division have equal precedence (they’re performed from left to right, as they appear in the expression), as do addition and subtraction.
Historical Development of Order of Operations
The standardized order of operations wasn’t always universally accepted. Throughout mathematical history, different notations and conventions existed. The current convention began to crystallize in the late 19th and early 20th centuries as mathematics education became more standardized and widespread.
Early mathematical texts often relied on careful positioning and spacing to indicate the intended order of calculations. The introduction of typewriters and printing presses necessitated more explicit rules about how to interpret mathematical expressions written in a linear format.
By the mid-20th century, the order of operations as we know it today had become the international standard, though slight variations in teaching methods persist across different countries and educational systems.
Why We Need Standardized Rules
Imagine if every mathematician, scientist, engineer, or economist followed their own personal preferences for calculating expressions. The resulting chaos would make collaboration impossible and lead to countless errors in everything from bridge designs to financial forecasts.
The standardized order of operations serves as a mathematical “grammar” that ensures everyone interprets expressions the same way. Like the rules of language, these mathematical conventions enable clear, unambiguous communication of numerical ideas.
Solving the 4×4-4÷4+4 Challenge Step-by-Step
The Correct Approach Using PEMDAS/BODMAS
Let’s solve the 4×4-4÷4+4 expression step by step, following the standard order of operations:
- Parentheses/Brackets: There are none in this expression, so we move to the next step
- Exponents/Orders: There are none in this expression, so we move to the next step
- Multiplication and Division (from left to right):
- First operation: 4×4 = 16
- Second operation: 4÷4 = 1
- Our expression is now: 16-1+4
- Addition and Subtraction (from left to right):
- First operation: 16-1 = 15
- Second operation: 15+4 = 19
- Final answer: 19
Following the standard order of operations, the correct answer to 4×4-4÷4+4 is 19.
Common Mistakes in Solving This Problem
Several common errors lead people to different answers:
Mistake 1: Performing operations strictly from left to right
If someone calculates from left to right without respecting the order of operations: 4×4-4÷4+4 → 16-4÷4+4 → 16-1+4 → 15+4 → 19
In this case, they accidentally get the right answer, but for the wrong reason!
Mistake 2: Assuming subtraction takes precedence over division
Some people might calculate: 4×4-4÷4+4 → 16-4÷4+4 → 16-(4÷4)+4 → 16-1+4 → 19
Again, they reach the correct answer, but by incorrectly grouping the operations.
Mistake 3: Performing addition before subtraction
Another common error: 4×4-4÷4+4 → 16-4÷4+4 → 16-4÷8 → 16-0.5 → 15.5
This is incorrect because division should be performed before addition.
Mistake 4: Incorrect application of PEMDAS
Some interpret PEMDAS to mean that multiplication comes before division and addition before subtraction, rather than understanding that multiplication/division have equal precedence, as do addition/subtraction: 4×4-4÷4+4 → 16-4÷4+4 → 16-1+4 → 16+(−1+4) → 16+3 → 19
This approach happens to yield the correct answer in this case, but it’s applying the rules incorrectly.
Verifying the Answer with Different Methods
To confirm our solution, let’s try different approaches:
Using Parentheses to Eliminate Ambiguity
We can add parentheses to clarify the order of operations: 4×4-4÷4+4 = (4×4)-(4÷4)+4 = 16-1+4 = 19
Calculator Verification
Most scientific calculators and programming languages that follow standard mathematical conventions will produce 19 when calculating 4×4-4÷4+4.
Cross-Checking with a Different Sequence
We can rearrange the expression while maintaining its mathematical meaning: 4×4+4-4÷4 = 16+4-1 = 20-1 = 19
All methods lead to the same answer: 19.
Why People Get Different Answers: The Great Mathematical Divide
Calculator Discrepancies and Technology Factors
Not all calculators are created equal. Basic four-function calculators typically perform calculations from left to right without considering the standard order of operations. When someone enters 4×4-4÷4+4 into such a calculator, they might get an incorrect result.
Even among scientific calculators, implementation differences can lead to variations. Some older models might have quirks in how they handle order of operations, especially with more complex expressions.
Smartphone calculator apps add another layer of complexity. Some default to simple calculation modes that don’t follow PEMDAS/BODMAS, while others have scientific modes that do. A person might get different answers depending on which app they use or which mode is active.
Educational Differences Around the World
While the mathematical convention for order of operations is internationally standardized, how it’s taught varies significantly:
- In some countries, the mnemonic PEMDAS might emphasize that multiplication always comes before division, even though they have equal precedence
- Some educational systems spend more time drilling order of operations than others
- The depth of explanation about why these rules exist varies widely
- In certain regions, teachers might focus more on using parentheses to avoid ambiguity rather than memorizing precedence rules
These educational differences mean that people from different countries or even different schools within the same country might approach the 4×4-4÷4+4 challenge with varying levels of understanding about how to solve it correctly.
Common Misunderstandings About PEMDAS/BODMAS
The most prevalent misunderstanding involves the letters M and D in PEMDAS and B in BODMAS. Many students incorrectly learn that:
- Multiplication always comes before division
- Addition always comes before subtraction
In reality, multiplication and division have equal precedence and should be performed from left to right as they appear in the expression. The same applies to addition and subtraction.
This subtle misinterpretation explains why many people confidently arrive at incorrect answers. They’re not making careless mistakes; they’re systematically applying a flawed understanding of the rules.
The Psychology Behind Mathematical Debates: Why Simple Math Goes Viral
The Dunning-Kruger Effect in Mathematical Confidence
The Dunning-Kruger effect—a cognitive bias where people with limited knowledge in a domain overestimate their competence—plays a significant role in mathematical debates. Most adults believe they’ve mastered elementary arithmetic operations, leading to high confidence in their answers to problems like 4×4-4÷4+4.
This overconfidence, combined with the surprising discovery that others disagree, creates the perfect environment for passionate debates. People are more likely to argue vehemently about topics where they feel certain of their knowledge but are confronted with contradictory views.
Confirmation Bias and Mathematical Reasoning
Once someone has calculated an answer, confirmation bias kicks in. They selectively seek information that supports their solution while dismissing contradictory evidence. If ten comments agree with their answer and ninety disagree, they’re likely to focus on the ten that align with their viewpoint.
This bias manifests in how people defend their answers—they might find creative ways to justify their calculation method or dismiss alternative approaches without truly engaging with them.
The Social Aspect of Mathematical Disagreements
Mathematical debates on social media tap into fundamental human desires for social validation and intellectual status. Publicly solving a math problem correctly signals intelligence, while discovering others have made a “simple” error can provide a sense of intellectual superiority.
The comment sections of viral math problems quickly transform into tribal battlegrounds with people forming allegiance groups around specific answers. The debate shifts from mathematical principles to social dynamics, with likes and supporting comments reinforcing people’s confidence in their positions.
The Virality Formula for Math Problems
The 4×4-4÷4+4 challenge and similar problems go viral precisely because they:
- Appear accessible: Anyone with basic math education feels qualified to attempt them
- Create genuine confusion: The ambiguity around order of operations leads to legitimate disagreement
- Trigger emotional responses: The combination of high confidence and contradictory answers generates frustration and surprise
- Invite participation: Commenting with an answer is a low-effort way to engage
- Drive shares: People share these challenges to test friends or prove others wrong
This perfect storm of factors makes simple-looking math problems some of the most engaging content on social media platforms, regularly generating millions of interactions.
Similar Viral Math Problems That Divided the Internet
The 8÷2(2+2) Controversy
Perhaps the most famous viral math problem is 8÷2(2+2). This expression creates even more controversy than our 4×4-4÷4+4 challenge because it introduces implied multiplication through parentheses.
Depending on whether one treats the implied multiplication 2(2+2) as having higher precedence than the explicit division, this problem yields either 1 or 16 as the answer. Even professional mathematicians and physicists have disagreed on the interpretation of this expression, highlighting how notation conventions can remain ambiguous even among experts.
6-1×0+2÷2 and Other Viral Calculations
Another viral problem, 6-1×0+2÷2, relies on a similar confusion about order of operations. Following PEMDAS correctly leads to the answer 6, but many people get 3.5 or other incorrect results.
These problems share common elements that make them confusing:
- A mix of operations with different precedence levels
- The absence of parentheses to clarify grouping
- Operations that appear in an order different from their precedence
Patterns in Viral Math Problems
Analyzing viral math problems reveals consistent patterns:
- They almost always involve order of operations
- They typically use single-digit numbers for accessibility
- They often include division and multiplication in sequence
- They rarely include exponents or other advanced operations
- They’re usually presented without contextual clues about their purpose
Content creators and social media pages have recognized these patterns and deliberately craft problems with these characteristics to maximize engagement. The formula is so effective that even old, recycled problems still generate massive engagement when reposted.
Educational Value: Learning Opportunities from Viral Math Debates
Classroom Applications of the 4×4-4÷4+4 Challenge
Despite their controversial nature, problems like the 4×4-4÷4+4 challenge offer valuable teaching opportunities:
- Demonstrating the importance of conventions: These problems show students why mathematical rules matter
- Encouraging critical thinking: Analyzing why different approaches lead to different answers develops analytical skills
- Practicing order of operations: Students can strengthen their understanding of PEMDAS/BODMAS through engaging examples
- Introducing mathematical communication: Discussing how to write unambiguous expressions teaches important documentation skills
Progressive educators have incorporated viral math problems into their curriculum, using them as entry points to deeper mathematical conversations. The controversy naturally engages students who might otherwise find order of operations dry or irrelevant.
Building Stronger Foundational Math Understanding
The confusion surrounding problems like 4×4-4÷4+4 highlights gaps in foundational mathematical understanding. Addressing these gaps can strengthen overall math education by:
- Emphasizing the “why” behind mathematical conventions
- Teaching students to recognize when an expression might be ambiguous
- Encouraging the use of parentheses to clarify intent
- Developing healthy skepticism about calculations without verification
For self-learners and students, confronting and resolving the confusion in these viral problems can build confidence in approaching more complex mathematical challenges.
Critical Thinking in the Age of Misinformation
The passionate debates around simple math problems mirror larger societal challenges with misinformation. Learning to:
- Verify information using reliable sources
- Recognize when one’s intuition might be leading astray
- Understand why different people might interpret the same information differently
- Approach disagreements with curiosity rather than defensiveness
These skills, practiced in the relatively low-stakes environment of mathematical debates, transfer to navigating more consequential disagreements in science, politics, and other domains.
Advanced Perspectives: Beyond Simple Answers
Mathematical Notation and Ambiguity in Different Contexts
While standard order of operations provides a clear answer to 4×4-4÷4+4 in educational contexts, professional mathematicians rarely write expressions in ways that could create ambiguity. In academic papers, textbooks, and professional work:
- Parentheses are used liberally to clarify intent
- Fractions are written vertically rather than using the division symbol
- Variables and functions are chosen to minimize confusion
- Expressions are broken into smaller, clearer components
The notation used in viral math problems like 4×4-4÷4+4 is primarily found in elementary and secondary education. Beyond that level, the focus shifts from memorizing precedence rules to communicating mathematical ideas clearly.
Different Conventions in Programming and Scientific Contexts
In programming languages, the rules for evaluating expressions may differ slightly from standard mathematical conventions:
# Python follows standard order of operations
result = 4*4-4/4+4 # Equals 19
// JavaScript also follows standard precedence
let result = 4*4-4/4+4; // Equals 19
However, some specialized notation systems in physics, engineering, or computer science might have domain-specific conventions that alter how expressions are interpreted. These specialized systems highlight that mathematical notation is ultimately a human invention designed to communicate ideas efficiently within specific contexts.
Professional Mathematicians’ Views on Viral Math Debates
When asked about viral math problems, professional mathematicians often express frustration at the framing of these debates. From their perspective:
- Clarity is paramount: In real mathematical work, expressing calculations unambiguously is more important than applying precedence rules
- Context matters: Mathematical notation only has meaning within agreed-upon conventions
- The goal is communication: Notation exists to communicate ideas precisely, not to create puzzles
Many mathematicians view these viral debates as missing the forest for the trees—focusing on applying memorized rules rather than understanding the purpose of mathematical notation as a communication tool.
Practical Applications: Order of Operations in the Real World
How Order of Operations Impacts Programming and Computing
In software development, understanding order of operations is crucial. Incorrect application of these rules can lead to bugs that are difficult to detect but cause significant problems. Consider a simple calculation for applying a discount to a product price:
let finalPrice = basePrice - discount * taxRate;
If a programmer misunderstands order of operations, they might expect the discount to be applied after the tax, when in fact the discount is multiplied by the tax rate first.
Similar issues arise in spreadsheet formulas, database queries, and data analysis scripts. The financial implications of such misunderstandings can be substantial in business applications.
Financial Calculations and Potential Costly Mistakes
In finance, applying operations in the wrong order can lead to significant monetary errors. For example, when calculating compound interest or investment returns, misunderstanding the order of applied rates and time periods can result in vastly different projections.
These errors aren’t merely academic—they affect real-world decisions about retirement planning, mortgage calculations, and business valuations. The difference between calculating (principal × (1 + rate)) versus (principal + principal × rate) might seem subtle but compounds dramatically over time.
Scientific Formulas and Precision Requirements
Scientific calculations demand extreme precision, making correct application of order of operations essential. From drug dosage calculations in medicine to trajectory calculations in aerospace engineering, following operation precedence correctly can be a matter of life and death.
In fields like chemistry, physics, and engineering, formulas often involve complex nested operations. Scientists rely on the standardized order of operations to ensure their calculations yield consistent, reliable results across different researchers and laboratories.
Teaching and Learning Strategies: Mastering Order of Operations
Effective Mnemonics Beyond PEMDAS
While PEMDAS and similar acronyms provide a starting point for learning order of operations, more nuanced approaches can prevent common misunderstandings:
- PEMA: Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right)
- Grouping symbols, Powers, Products and Quotients, Sums and Differences: A longer but more precise description
- Visual hierarchies: Using vertical arrangements to show operation precedence levels
Some educators have found success with storytelling approaches, creating characters or scenarios that help students remember the correct sequence and the equal precedence of certain operations.
Visualization Techniques for Understanding Operation Precedence
Visual aids can make abstract order of operations more concrete:
- Color-coding different operations based on their precedence level
- Nested boxes showing which calculations should be performed first
- Tree diagrams illustrating the hierarchical structure of expressions
- Animation sequences demonstrating the step-by-step evaluation process
These visualization techniques help students see the structure of expressions rather than just mechanically applying rules.
Practice Exercises That Build Confidence
Effective practice goes beyond solving isolated problems. Comprehensive exercises should include:
- Creating expressions with given answers to reinforce understanding
- Identifying errors in incorrectly worked examples
- Adding parentheses to ambiguous expressions to clarify intent
- Translating word problems into mathematical expressions
- Explaining reasoning behind each step of a solution
This varied approach builds deeper understanding than simply drilling PEMDAS exercises.
Conclusion: The Value of Mathematical Clarity
What the 4×4-4÷4+4 Challenge Teaches Us
The 4×4-4÷4+4 challenge offers several valuable lessons:
- Mathematical conventions matter: Without agreed-upon rules, even simple expressions become ambiguous
- Clarity should be prioritized: Using parentheses to make intent explicit is better than relying on precedence rules
- Digital literacy includes calculator awareness: Understanding how different calculators interpret expressions is increasingly important
- Viral confusion can spark learning: The controversy around these problems creates opportunities for deeper understanding
These seemingly trivial math challenges reveal how fundamental mathematical communication is to our interconnected, technology-driven world.
The Broader Implications for Mathematical Literacy
In an era where data literacy and computational thinking are essential skills, the confusion surrounding problems like 4×4-4÷4+4 highlights the need for stronger mathematical foundations.
As automation and AI increasingly handle routine calculations, human value lies in understanding mathematical principles and communicating them clearly. The ability to write unambiguous mathematical expressions and interpret them correctly becomes more, not less, important in a technology-driven society.
Final Thoughts on the 4×4-4÷4+4 Challenge
The next time you encounter the 4×4-4÷4+4 challenge or a similar viral math problem, consider it an opportunity rather than a frustration. These problems remind us that mathematics isn’t just about reaching the right answer—it’s about clear communication, consistent conventions, and critical thinking.
And if you’re wondering, the answer is indeed 19. But more important than the answer itself is understanding why it’s 19, how others might reach different conclusions, and how to express mathematical ideas in ways that minimize confusion.
In mathematics, as in life, clarity is a virtue worth cultivating.
FAQs About the 4×4-4÷4+4 Challenge
What is the correct answer to 4×4-4÷4+4?
Following standard order of operations (PEMDAS/BODMAS), the correct answer is 19. First, perform multiplication and division from left to right (4×4=16, 4÷4=1), resulting in 16-1+4, then perform addition and subtraction from left to right (16-1=15, 15+4=19).
Why do calculators sometimes give different answers?
Basic calculators often perform operations sequentially from left to right without following order of operations, leading to incorrect results. Scientific calculators and most smartphone calculator apps in scientific mode follow standard order of operations and will give the correct answer of 19.
What is PEMDAS/BODMAS and why does it matter?
PEMDAS (Parentheses, Exponents, Multiplication/Division, Addition/Subtraction) and BODMAS (Brackets, Orders, Division/Multiplication, Addition/Subtraction) are acronyms that help remember the standard order of operations in mathematics. These conventions ensure everyone interprets mathematical expressions consistently.
How can I avoid making mistakes with order of operations?
Use parentheses to clarify your intent, work step-by-step, write out intermediate results, and remember that multiplication/division have equal precedence (work left to right), as do addition/subtraction (also left to right).
Are there situations where order of operations doesn’t apply?
In standard mathematics, order of operations always applies. However, some programming languages, specialized notation systems, or context-specific conventions might alter these rules. When in doubt, use parentheses to make your intention explicit.
How can teachers use the 4×4-4÷4+4 challenge in education?
Teachers can use this challenge to demonstrate the importance of order of operations, encourage critical thinking about mathematical conventions, practice step-by-step calculation, and discuss how mathematical disagreements can be resolved through reference to established principles.
Where did the standard order of operations come from?
The standardized order of operations evolved gradually as mathematical notation developed, becoming more formalized in the late 19th and early 20th centuries. It was established to ensure mathematical expressions could be interpreted consistently across different contexts and cultures.
Why do so many people get different answers to the same math problem?
Different answers arise from misunderstanding the precedence of operations (particularly thinking multiplication always comes before division), performing calculations strictly left to right, using calculators that don’t follow standard order of operations, or making simple arithmetic errors during calculation.
Bubble Pop, Memory Match, Endless Alphabet & Panda Pop
In today’s digital age, mobile games have become more than just a source of entertainment—they’ve evolved into powerful learning tools that can significantly impact a child’s cognitive development. Among the vast array of free games available, four stand out for their engaging gameplay and educational benefits: Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game. These free learning games offer more than just fun; they provide crucial developmental advantages that can enhance a child’s memory, problem-solving skills, and overall cognitive abilities.
The Hidden Educational Power of Mobile Games
Before diving into the specifics of each game, it’s important to understand why these seemingly simple entertainment apps have garnered attention from parents, educators, and child development experts alike. The convergence of play and learning creates an environment where children absorb knowledge without the pressure or boredom sometimes associated with traditional learning methods.
Mobile games tap into the brain’s reward system, releasing dopamine when players accomplish goals or overcome challenges. This natural reward mechanism creates a positive association with learning, encouraging children to continue engaging with educational content. It’s a perfect example of how free courses of learning can be embedded into everyday play.
Bubble Pop Game: More Than Just Popping Bubbles
What Makes Bubble Pop Games Special?
Bubble Pop Game variants have dominated the casual mobile gaming scene for years, and for good reason. The premise is simple: players match colorful bubbles to clear them from the screen. However, beneath this straightforward concept lies a complex web of cognitive benefits.
Cognitive Benefits for Growing Minds
When children engage with Bubble Pop Game mechanics, they’re actually exercising several critical mental functions:
- Color Recognition and Matching: The fundamental gameplay requires identifying and matching colors, a basic cognitive skill that forms the foundation for more complex learning.
- Strategic Thinking: As levels progress, children must develop strategies to clear bubbles efficiently, promoting forward-thinking and planning.
- Spatial Awareness: Bubble placement requires understanding of how objects interact in a defined space—a crucial developmental skill.
- Hand-Eye Coordination: The timing and precision needed to aim and shoot bubbles enhances fine motor skills and coordination.
Top Bubble Pop Games for Different Age Groups
For toddlers (ages 2-4):
- Bubble Pop Baby Games: Simplified mechanics with bright colors and rewarding sound effects
- Baby Bubble Pop: Features large, easy-to-tap bubbles with animal sounds
For preschoolers (ages 4-6):
- Bubble Shooter for Kids: Introduces basic matching concepts with guided play
- Tiny Bubbles: Teaches color mixing principles alongside bubble popping
For elementary school children (ages 6-12):
- Bubble Witch 3 Saga: Adds narrative elements while maintaining the educational core
- Bubble Shooter Rainbow: Incorporates more complex color patterns and strategies
Real-World Skills Developed Through Bubble Games
The skills cultivated through Bubble Pop Game play extend beyond the screen:
- Pattern Recognition: Essential for mathematics and reading
- Decision-Making Under Pressure: Valuable for everyday problem-solving
- Resilience Through Repetition: Learning to persist despite challenges
Memory Match Game: Training Young Brains for Information Retention
The Science Behind Memory Games
Memory Match Game formats leverage the psychological principle of spaced repetition—a learning technique that involves reviewing information at gradually increasing intervals. This method has been proven to enhance long-term memory retention.
How Memory Match Enhances Cognitive Development
The simple act of flipping virtual cards and remembering their positions engages multiple cognitive processes:
- Working Memory Exercise: Children must hold information temporarily while processing other cards.
- Visual Processing Enhancement: Recognizing and remembering images strengthens visual discrimination abilities.
- Attention Span Extension: The need to focus on the game naturally increases concentration duration.
- Conceptual Categorization: More advanced Memory Match Games that group cards by themes help children develop categorization skills.
Memory Match Variations for Different Learning Styles
Visual learners:
- Photo Memory Match: Uses realistic images of objects, animals, or places
- Shape and Color Match: Focuses on geometric patterns and color combinations
Auditory learners:
- Sound Memory Match: Pairs identical sounds instead of images
- Musical Memory: Matches musical instruments or notes
Kinesthetic learners:
- Action Memory Match: Combines physical movements with card matching
- Touch-Sensitive Memory Games: Provides tactile feedback when matches are made
Memory Match as a Tool for Academic Skills
The versatility of Memory Match Game formats makes them ideal vehicles for academic content:
- Alphabet Matching: Pairs uppercase and lowercase letters
- Numerical Matching: Connects numbers with the corresponding quantity of objects
- Vocabulary Building: Matches words with their visual representations
- Foreign Language Acquisition: Pairs words in different languages
Creating Custom Memory Match Experiences
Many free learning apps allow for customization, enabling parents and educators to create personalized Memory Match Game experiences that target specific learning objectives:
- Family Photo Memory: Using familiar faces to engage younger children
- Current Curriculum Match: Incorporating content from school lessons
- Interest-Based Matching: Focusing on topics that excite the individual child
Endless Alphabet Game: Transforming Literacy Learning
Revolutionary Approach to Letter Recognition
The Endless Alphabet Game has transformed how children approach the fundamentally important task of learning letters and words. Instead of rote memorization, this innovative app brings letters to life through animation and interactivity.
The Mechanics of Playful Literacy
The genius of the Endless Alphabet Game lies in its multi-sensory approach:
- Interactive Letter Placement: Children drag animated characters (letters) into their correct positions.
- Phonetic Sound Reinforcement: Each letter makes its phonetic sound when touched, reinforcing sound-symbol relationships.
- Word Animation: Completed words trigger humorous animations that illustrate the word’s meaning.
- Vocabulary Expansion: The game introduces words beyond the typical preschool vocabulary, enriching language exposure.
Developmental Milestones Supported by Alphabetic Play
The Endless Alphabet Game supports multiple developmental milestones:
- Pre-Reading Skills: Foundational phonemic awareness and letter recognition
- Fine Motor Development: Precision required for letter dragging and placement
- Vocabulary Acquisition: Exposure to rich, varied vocabulary with context
- Sequential Processing: Understanding the correct order of letters in words
Beyond Basic Literacy: Advanced Features
The educational depth of the Endless Alphabet Game extends beyond basic letter recognition:
- Word Categorization: Words are often thematically grouped, building conceptual understanding
- Contextual Learning: Animations provide context clues about word meanings
- Morphological Awareness: Exposure to prefixes, suffixes, and word roots in more advanced words
- Pronunciation Models: Clear audio pronunciation models support speech development
Integrating Endless Alphabet into Daily Learning Routines
The Endless Alphabet Game’s flexibility makes it ideal for integration into daily routines:
- Morning Alphabet Practice: Starting the day with a few words builds positive learning momentum
- Travel Companion: Turning transit time into productive learning opportunities
- Bedtime Wind-Down: A calmer alternative to more stimulating games before sleep
- Vocabulary Boost: Targeted use to support words encountered in books or conversation
Panda Pop Game: Strategic Thinking in Disguise
The Captivating World of Panda Pop
While the cute panda characters and colorful bubbles might seem purely entertainment-focused, Panda Pop Game mechanics incorporate sophisticated problem-solving elements that challenge developing minds.
Cognitive Challenges Hidden in Cute Packaging
The Panda Pop Game offers several cognitive challenges:
- Physics-Based Problem Solving: Understanding how bubbles will interact based on trajectory and momentum.
- Resource Management: Limited bubbles require thoughtful use of available resources.
- Goal Prioritization: Multiple objectives within levels teach prioritization skills.
- Adaptive Strategy Development: Changing level designs require flexible thinking and strategy adaptation.
Mathematical Concepts Embedded in Gameplay
The Panda Pop Game subtly incorporates mathematical thinking:
- Geometric Principles: Angle calculation for optimal bubble placement
- Probability Assessment: Predicting likely outcomes of various moves
- Numerical Progression: Understanding level progression and scoring systems
- Set Theory Basics: Grouping similar items (bubbles of the same color)
Problem-Solving Approaches Developed Through Play
Regular engagement with Panda Pop Game challenges promotes several problem-solving approaches:
- Trial and Error Learning: Testing strategies and learning from results
- Pattern Recognition: Identifying recurring level designs and optimal solutions
- Decomposition Skills: Breaking complex levels into manageable sections
- Algorithmic Thinking: Developing step-by-step approaches to challenges
Social and Emotional Learning Through Panda Pop
Beyond cognitive benefits, the Panda Pop Game format offers opportunities for social-emotional development:
- Frustration Tolerance: Learning to persist through challenging levels
- Achievement Recognition: Celebrating milestones and accomplishments
- Community Participation: Many versions include team challenges or friend interactions
- Emotional Regulation: Managing the excitement of success and disappointment of setbacks
The Intersection of Free Games and Quality Learning
Breaking Down the Cost Barrier to Education
One of the most significant advantages of games like Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game is their accessibility. These free games demolish financial barriers to quality learning experiences, democratizing educational content.
The Free Learning Revolution in Mobile Gaming
The availability of high-quality free learning opportunities through gaming represents a revolution in educational access:
- Universal Access: Children across socioeconomic backgrounds can access identical learning content.
- Supplemental Education: Free games provide additional learning opportunities beyond formal education.
- Differentiated Learning Paths: Various game types address different learning styles and needs without additional cost.
- Continuous Content Updates: Many free games regularly update content, providing fresh learning challenges.
Evaluating Quality in Free Educational Games
Not all free games offer equal educational value. Parents and educators should consider these factors when selecting free learning games:
- Developmental Appropriateness: Content matched to the child’s current abilities with room for growth
- Learning Integration: Educational elements seamlessly woven into enjoyable gameplay
- Engagement Sustainability: Ability to maintain interest beyond novelty period
- Progress Measurement: Features that track development and mastery
- Ethical Design: Absence of manipulative monetization tactics or inappropriate content
Monetization Models and Educational Integrity
Understanding how free games are monetized helps assess their educational integrity:
- Ad-Supported Models: Consider frequency, content, and placement of advertisements
- Freemium Approaches: Evaluate whether core educational content is truly accessible without payment
- Data Collection Practices: Review privacy policies regarding information gathered from young users
- Premium Content Balance: Determine if non-paying users still receive substantial educational value
Integrating Educational Games into Balanced Development
Creating a Holistic Digital Learning Environment
While games like Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game offer tremendous benefits, they work best as part of a balanced approach to child development.
Screen Time Guidelines for Educational Gaming
Research-based recommendations for educational game time include:
- Age-Appropriate Limits: Following pediatric guidelines for total screen time
- Co-Play Sessions: Adult participation enhances learning transfer
- Natural Stopping Points: Respecting attention span limitations rather than enforcing arbitrary time limits
- Balance Indicators: Monitoring for signs that digital play is displacing other essential activities
Complementary Non-Digital Activities
To maximize the benefits of educational games, pair them with related offline activities:
For Bubble Pop Game:
- Physical bubble-blowing and popping
- Color sorting games with household objects
- Target practice activities for hand-eye coordination
For Memory Match Game:
- Traditional physical memory card games
- Scavenger hunts with memory elements
- “What’s missing?” observation games
For Endless Alphabet Game:
- Letter formation with clay or finger paints
- Sound scavenger hunts (finding objects that start with specific sounds)
- Storytelling incorporating newly learned vocabulary
For Panda Pop Game:
- Strategy board games appropriate for age
- Building challenges with blocks or construction toys
- Puzzle-solving activities of increasing complexity
Monitoring and Supporting Progress
Effective integration of educational games involves ongoing assessment and support:
- Observational Assessment: Noting which skills seem to be developing through gameplay
- Conversation Integration: Discussing game concepts during everyday activities
- Challenge Calibration: Ensuring the child is appropriately challenged but not frustrated
- Interest Expansion: Using game topics as springboards to broader learning
The Future of Educational Gaming
Emerging Technologies Enhancing Learning Games
The educational potential of games like Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game continues to expand with technological advancements:
- Augmented Reality Integration: Blending physical environments with digital game elements
- Adaptive Learning Algorithms: Customizing difficulty and content based on individual performance
- Voice Recognition Components: Adding spoken language elements to gameplay
- Cross-Platform Continuity: Seamless learning experiences across multiple devices
Personalized Learning Pathways
The future of educational gaming points toward increasingly personalized experiences:
- Learning Style Adaptation: Games that detect and adjust to individual learning preferences
- Progress-Based Content Delivery: Just-in-time learning materials based on demonstrated mastery
- Interest-Driven Branching: Content paths that follow the child’s curiosity
- Strength and Challenge Identification: Analytics that identify areas of proficiency and growth opportunity
Research-Informed Game Development
As researchers continue to study the impact of games on learning, we can expect developments in:
- Cognitive Load Optimization: Game design that maximizes learning while minimizing mental fatigue
- Transfer Effect Enhancement: Features that improve the application of game-learned skills to real-world situations
- Attention Management Mechanisms: Elements that support healthy attention development rather than exploiting attention vulnerabilities
- Social Learning Integration: Multiplayer educational experiences that leverage peer learning effects
Conclusion: The Lasting Impact of Educational Gaming
The seemingly simple activities found in Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game represent a profound shift in how we approach early childhood education. These free games and free learning opportunities remove barriers to quality educational experiences while embedding crucial developmental support in engaging play.
As parents, educators, and caregivers navigate the complex landscape of children’s media, these educational games stand out as valuable tools that respect both the child’s need for enjoyment and their capacity for growth. The skills developed—from memory enhancement and strategic thinking to literacy foundations and problem-solving approaches—establish cognitive frameworks that support lifelong learning.
The true power of these games lies not just in their individual benefits but in their collective contribution to a child’s developing mind. When thoughtfully integrated into a balanced approach to learning and development, Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game become more than just free courses in disguise—they become catalysts for curiosity, confidence, and cognitive growth.
By understanding the educational mechanisms at work behind the colorful interfaces and engaging gameplay, adults can make informed choices about which games will best support specific developmental needs while providing the joy and engagement that makes learning stick. In the expanding universe of digital media competing for children’s attention, these educational games represent some of the brightest stars—illuminating paths to knowledge through the simple, universal language of play.
FAQs About Educational Mobile Games
Are free games as educational as paid options?
Many free games offer educational value comparable to paid alternatives. The key factors are the game’s design principles and learning integration rather than price point. Some free games supported by reputable educational organizations may actually offer superior learning experiences compared to certain paid options.
How much time should children spend on educational games daily?
The American Academy of Pediatrics recommends that children ages 2-5 limit screen time to 1 hour per day of high-quality programming, while children 6 and older should have consistent limits on screen time in general. Within these guidelines, educational games like Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game can form a valuable portion of the allocated time.
At what age should children start playing educational mobile games?
Most developmental experts recommend limiting screen exposure for children under 18-24 months, except for video chatting. Between ages 2-5, carefully selected educational games with adult participation can be introduced in limited durations. The games discussed in this article vary in appropriate starting ages, with simplified versions of Memory Match Game potentially suitable for children as young as 2, while Panda Pop Game might be more appropriate for children 4 and older.
How can I tell if a game is truly educational?
Look for games that:
- Clearly state learning objectives
- Integrate learning naturally into gameplay rather than as separate “educational” sections
- Provide appropriate challenges that adapt to skill level
- Offer meaningful feedback beyond simple “right/wrong” responses
- Avoid excessive rewards for minimal effort
- Come from developers with educational expertise or partnerships
Can these games help children with learning differences?
Yes, games like Bubble Pop Game, Memory Match Game, Endless Alphabet Game, and Panda Pop Game often provide multi-sensory learning experiences that can benefit children with various learning differences. The self-paced nature, immediate feedback, repetition without judgment, and multiple modes of interaction make them valuable tools for diverse learners. However, games should be selected with the specific child’s needs in mind, and it’s often helpful to consult with educational specialists for recommendations.