Understanding 3D Shapes: A Comprehensive Guide
3D shapes are all around us, from the simplest box to the most complex architectural structures. They make up the world we live in and have numerous applications in mathematics, engineering, design, and art. In this comprehensive blog post, we will explore the concept of 3D shapes, their different types, and various ways they are utilized. We will also touch on popular keywords like “2D 3D shape testing for adults on YouTube video” and “custom 3D printed shapes,” making this guide SEO-rich and informative.
What Are 3D Shapes?
3D shapes, or three-dimensional shapes, are geometric figures that have three dimensions: length, width, and height. These shapes have volume and occupy space, which makes them distinct from 2D shapes, which are flat and only have two dimensions. Common 3D geometric shapes include cubes, spheres, cylinders, cones, pyramids, and prisms.
Names of 3D Shapes and Their Characteristics
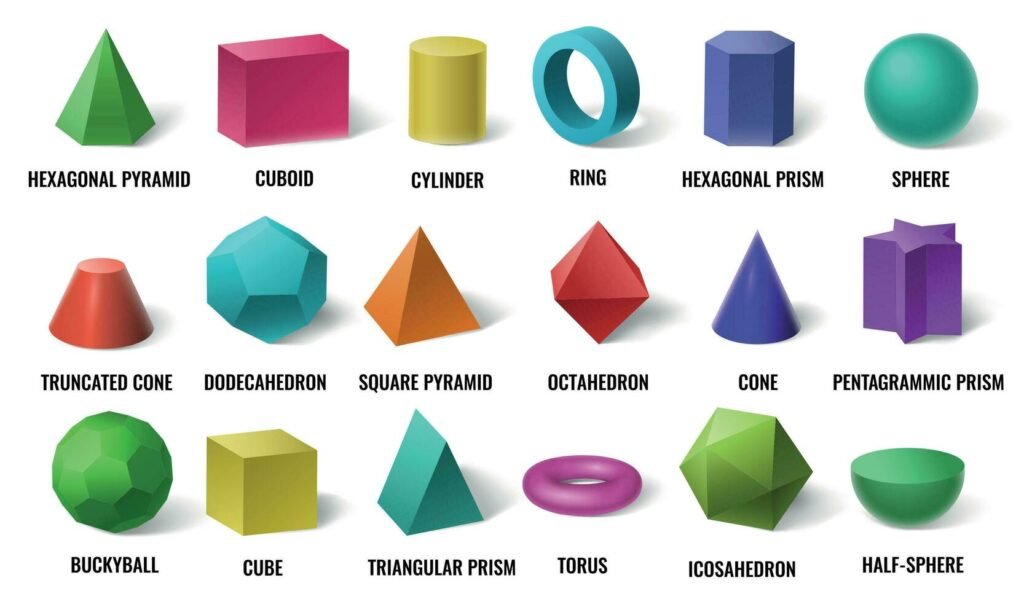
Understanding the different types of 3D shapes and their names is essential for studying geometry. Here is a list of some common 3D shapes with their names and properties:
Cube: A six-faced shape with all sides equal and faces that are squares.
Sphere: A perfectly round shape with no edges or vertices.
Cylinder: A shape with two parallel circular faces connected by a curved surface.
Pyramid: A shape with a polygonal base and triangular faces that meet at a single point, called the apex.
Cone: A shape with a circular base and a pointed apex, commonly referred to as a cone 3D shape.
Prism: A shape with two parallel, identical bases connected by rectangular faces.
Torus: Often described as a 3D ring shape, it looks like a donut.
These 3D shapes and names are crucial for understanding the various applications of geometry in everyday life.
Drawing and Understanding 3D Shapes
Drawing 3D shapes can help in visualizing complex concepts, whether you are an artist or a student studying geometry. The act of drawing shapes in 3D involves perspective, shading, and understanding the geometry of the object. For example, when drawing of 3D shapes is called for in school assignments, you might be asked to sketch a cube with its edges and vertices clearly defined.
If you’re curious about how to sketch 3D shapes, consider checking out tutorials on YouTube. There are numerous 2D 3D shape testing for adults on YouTube videos that can help you practice drawing skills and test your understanding of geometric shapes.
3D Shapes and Printing
Custom 3D printed shapes have become increasingly popular, thanks to advancements in 3D printing technology. You can create anything from a simple carrot-shaped bookmark 3D print to intricate models of 3D geometric shapes for educational purposes or decoration.
3D printing is an excellent way to bring digital models to life. Programs like Shaper 3D and Matlab are often used to create complex models that can be printed. For example, you can use Matlab to take a 2D slice of a 3D alpha shape and then transform it into a printable object.
Formulas and Calculations for 3D Shapes
When dealing with 3D shapes, understanding the associated formulas is key. Here are some important formulas related to 3D shapes geometry:
Volume of 3D Shapes: Each 3D shape has a unique formula for calculating its volume. For instance, the volume of a cube is V = a³, where a is the length of a side.
Surface Area of 3D Shapes: Surface area represents the total area of all the faces of a 3D shape. The formula for the surface area of a cube is A = 6a².
Calculus and 3D Shapes: In calculus, you might encounter problems involving formulas for 3D shapes, such as calculating the volume of a solid using integration.
For advanced learners, worksheets like Match the Equation to the Graph 3D Shapes 13.6 Worksheet can provide an excellent challenge for practicing how different 3D equations relate to their visual representations.
Faces, Edges, and Vertices of 3D Shapes
Every 3D shape can be described by its faces, edges, and vertices:
Faces are the flat surfaces that make up the boundaries of a 3D shape.
Edges are the lines where two faces meet.
Vertices are the points where edges meet.
For example, a cube has 6 faces, 12 edges, and 8 vertices. Understanding the relationship between faces, edges, and corners of 3D shapes is fundamental in geometry. This concept is particularly useful for students learning about 3D shapes in kindergarten or for adults revisiting the basics.
Interactive Learning: 3D Shapes Song and Anchor Charts
Learning about 3D shapes can be fun, especially for young children. Songs like the 3D shapes song and visual aids such as 3D shapes anchor charts help children learn 3D shapes with their names in an engaging way. These resources are especially popular in classrooms for teaching 2D and 3D shapes to young students.
2D vs. 3D Shapes
2D shapes and 3D shapes differ in that 2D shapes have only length and width, while 3D shapes have length, width, and height. Common 2D shapes include circles, squares, and triangles, while 3D shapes include spheres, cubes, and pyramids.
In advanced applications, you may encounter 2D shapes into 3D shapes, which involves taking a flat 2D shape and rotating or extruding it to create a 3D object. This concept is also a key part of geometry 3D shapes studies.
Cross Sections and Nets of 3D Shapes
A cross section of a 3D shape is the shape you get when you cut straight through a 3D object. Cross sections can provide valuable insights into the internal structure of the shape. Additionally, nets of 3D shapes are used to visualize how a 3D object can be unfolded into a 2D representation. For example, a cube’s net consists of six connected squares that, when folded, form the cube.
Frequently Asked Questions about 3D Shapes
Q1: What are 3D shapes and their types?
3D shapes are geometric figures that have three dimensions: length, width, and height. Common types include cubes, spheres, cylinders, cones, and pyramids.
Q2: How do you draw 3D shapes?
To draw 3D shapes, start by sketching the basic 2D outline and then add depth by drawing additional lines that show the third dimension. Practice with drawing 3D shapes worksheets can help improve this skill.
Q3: What are the formulas for calculating the volume of 3D shapes?
The volume of 3D shapes can be calculated using specific formulas. For example, the volume of a cone is given by V = (1/3)πr²h, where r is the radius of the base, and h is the height.
Q4: What are the differences between 2D and 3D shapes?
2D shapes are flat and only have length and width, while 3D shapes have depth in addition to length and width. This makes 3D shapes capable of holding volume.
Q5: What are faces, edges, and vertices?
Faces are the flat surfaces of a 3D shape. Edges are the lines where two faces meet, and vertices are the points where edges come together.
Conclusion
3D shapes are an integral part of both our physical world and the field of geometry. From custom 3D printed shapes to drawing 3D shapes, understanding these concepts can open doors to many applications in design, architecture, education, and beyond. Whether you are testing your skills through a 2D 3D shape testing for adults on YouTube video or exploring advanced concepts like volume formulas for 3D shapes, there is always something new to learn about these fascinating geometric figures.
Frequently Asked Questions about 3D Shapes:
What are 3D shapes and their types? 3D shapes have three dimensions: length, width, and height. Common types include cubes, spheres, cones, pyramids, cylinders, and prisms.
How do you draw 3D shapes? Drawing 3D shapes involves sketching the basic 2D shape and adding depth using parallel or angled lines to represent the third dimension.
What are the formulas for calculating the volume of 3D shapes? Common formulas include:
- Cube: V=a3V = a^3V=a3
- Sphere: V=43πr3V = \frac{4}{3} \pi r^3V=34πr3
- Cylinder: V=πr2hV = \pi r^2 hV=πr2h
- Cone: V=13πr2hV = \frac{1}{3} \pi r^2 hV=31πr2h
- Rectangular Prism: V=l×w×hV = l \times w \times hV=l×w×h
What are the differences between 2D and 3D shapes? 2D shapes have only length and width (e.g., square), while 3D shapes have length, width, and height (e.g., cube).
What are faces, edges, and vertices?
- Faces: The flat surfaces of a 3D shape.
- Edges: The lines where two faces meet.
- Vertices: The points where edges meet.
How are custom 3D printed shapes made? Custom 3D shapes are created using 3D modeling software and printed layer by layer using a 3D printer.
What is the difference between 2D shapes into 3D shapes and cross sections of 3D shapes? Transforming 2D shapes into 3D shapes involves adding depth. A cross-section of a 3D shape is a 2D slice through the object.
How do faces, edges, and vertices relate in a cube? A cube has 6 faces, 12 edges, and 8 vertices. The edges are where the faces meet, and the vertices are the points where the edges meet.
What tools can help in visualizing 3D shapes? Tools like CAD software, 3D modeling apps, and physical models help visualize 3D shapes.
How can I calculate the surface area of different 3D shapes? Each shape has its own formula:
- Cube: A=6a2A = 6a^2A=6a2
- Sphere: A=4πr2A = 4 \pi r^2A=4πr2
- Cylinder: A=2πr(h+r)A = 2\pi r(h + r)A=2πr(h+r)
- Cone: A=πr(r+h2+r2)A = \pi r(r + \sqrt{h^2 + r^2})A=πr(r+h2+r2)
What are some examples of 3D shapes in real life? Examples include dice (cube), basketballs (sphere), cans (cylinder), and pyramids (pyramid).
What is a torus, and how is it different from a sphere? A torus is a donut-shaped object, while a sphere is a perfectly round 3D shape. A torus has a hole in the center, unlike a sphere.
How do you calculate the volume of a cylinder? The formula for the volume of a cylinder is V=πr2hV = \pi r^2 hV=πr2h, where rrr is the radius and hhh is the height.
What is the formula for the surface area of a sphere? The formula for the surface area of a sphere is A=4πr2A = 4 \pi r^2A=4πr2, where rrr is the radius.
How are 3D shapes used in engineering? 3D shapes are used in engineering for designing objects, analyzing structures, and simulating real-world scenarios.
What are cross sections of 3D shapes? A cross-section is the intersection of a 3D shape with a plane, revealing a 2D shape.
What is a 3D shape net? A 3D shape net is a two-dimensional arrangement of all faces of a 3D shape that can be folded to form the 3D object.
How do you use nets to understand 3D shapes? Nets help visualize how 2D faces come together to form a 3D shape.
How are 3D shapes used in architecture? Architects use 3D shapes to design buildings and structures, taking into account space, stability, and aesthetics.
How do you find the volume of a cone? The formula for the volume of a cone is V=13πr2hV = \frac{1}{3} \pi r^2 hV=31πr2h, where rrr is the radius of the base and hhh is the height.
What is a 3D geometric shape? A 3D geometric shape is any solid object with three dimensions, such as cubes, pyramids, cylinders, and spheres.
How do 3D shapes differ from 2D shapes in terms of properties? 3D shapes have volume, while 2D shapes have only area. Additionally, 3D shapes have faces, edges, and vertices, unlike 2D shapes.
What is the significance of edges in a 3D shape? Edges define the boundaries where two faces meet and contribute to the overall shape and structure.
What are vertices in a 3D shape? Vertices are the corners where edges of a 3D shape meet, playing a critical role in the shape’s structure.
How are 3D shapes helpful in understanding spatial relationships? 3D shapes provide insight into how objects occupy space and interact in the real world, helping with navigation and design.
How do you draw a cube in 3D? To draw a cube, sketch two overlapping squares and connect their corresponding corners with straight lines.
What are some examples of 3D printing projects? Examples include custom jewelry, prototypes, architectural models, and educational tools.
How does a 3D printer create a 3D shape? A 3D printer builds objects layer by layer using materials like plastic or resin, guided by digital models.
How do you calculate the volume of a pyramid? The formula is V=13×Base Area×HeightV = \frac{1}{3} \times \text{Base Area} \times \text{Height}V=31×Base Area×Height.
What are the main features of a prism? A prism has two parallel, congruent bases and rectangular faces connecting the sides of the bases.
What is the difference between a cube and a cuboid? A cube has all sides equal, while a cuboid has different lengths, widths, and heights.
How do you sketch a 3D pyramid? Draw a base (square or triangle) and then add lines from the corners to a single point above the base.
How do you determine the number of faces on a 3D shape? The number of faces is determined by counting the flat surfaces that make up the 3D shape.
How can you create a 3D model using software? Use 3D modeling software like Blender, Tinkercad, or AutoCAD to design shapes with precise dimensions and features.
What is the relationship between edges and vertices in a pyramid? In a pyramid, edges are the lines connecting vertices, and the vertices are points where these edges meet.
What are some practical applications of 3D shapes? Applications include architecture, engineering, gaming, animation, and manufacturing.
How do you draw a 3D sphere? Start by drawing a circle, and then add shading or depth lines to create the illusion of three-dimensionality.
What is a carrot-shaped bookmark 3D print? It is a 3D-printed object shaped like a carrot, used as a decorative bookmark.
How do faces, edges, and vertices relate in a cone? A cone has one circular face, one curved edge, and one vertex (the tip).
What is the significance of 3D shapes in computer graphics? 3D shapes form the basis of models in computer graphics, used to create realistic objects in video games, movies, and simulations.
How do you use Matlab to take a 2D slice of a 3D alpha shape? In Matlab, functions like
alphaShapeandsliceallow you to visualize a 2D cross-section of a 3D shape.How do you identify the shape of a cross-section? The shape of a cross-section depends on the plane of the cut and the geometry of the 3D object.
How can 3D shapes be used in mathematics? They are used to study geometry, spatial relationships, and calculus applications like volume and surface area calculations.
What is the formula for the volume of a torus? The volume of a torus is V=2π2Rr2V = 2 \pi^2 R r^2V=2π2Rr2, where RRR is the distance from the center of the tube to the center of the torus, and rrr is the radius of the tube.
How can you create 3D shapes using Shaper 3D software? Shaper 3D allows users to sketch 2D shapes and extrude them into 3D, modify dimensions, and create complex models.
What are some fun ways to teach 3D shapes to children? Use interactive games, 3D modeling apps, physical models, and fun exercises like drawing or building shapes with clay.
How do you calculate the surface area of a cone? The surface area of a cone is A=πr(r+l)A = \pi r(r + l)A=πr(r+l), where rrr is the radius of the base and lll is the slant height.
What are the applications of 3D shapes in design? 3D shapes are used in product design, architectural modeling, graphic design, and more to create functional and aesthetic objects.
What are the differences between 3D shapes and solids? Solids are a subset of 3D shapes, referring specifically to objects that occupy space fully (not hollow).
How do you calculate the volume of a rectangular prism? The volume is V=l×w×hV = l \times w \times hV=l×w×h, where lll is the length, www is the width, and hhh is the height.